Fractions: Adding, Subtracting, Multiplying and Dividing

What is a Fraction?

A fraction is just something that has been cut up into equal parts. Imagine if you have a candy bar and you cut it up into 4 equal pieces—if you want to share that candy bar with three people, you would be giving away 3/4 (three-fourths) of it.

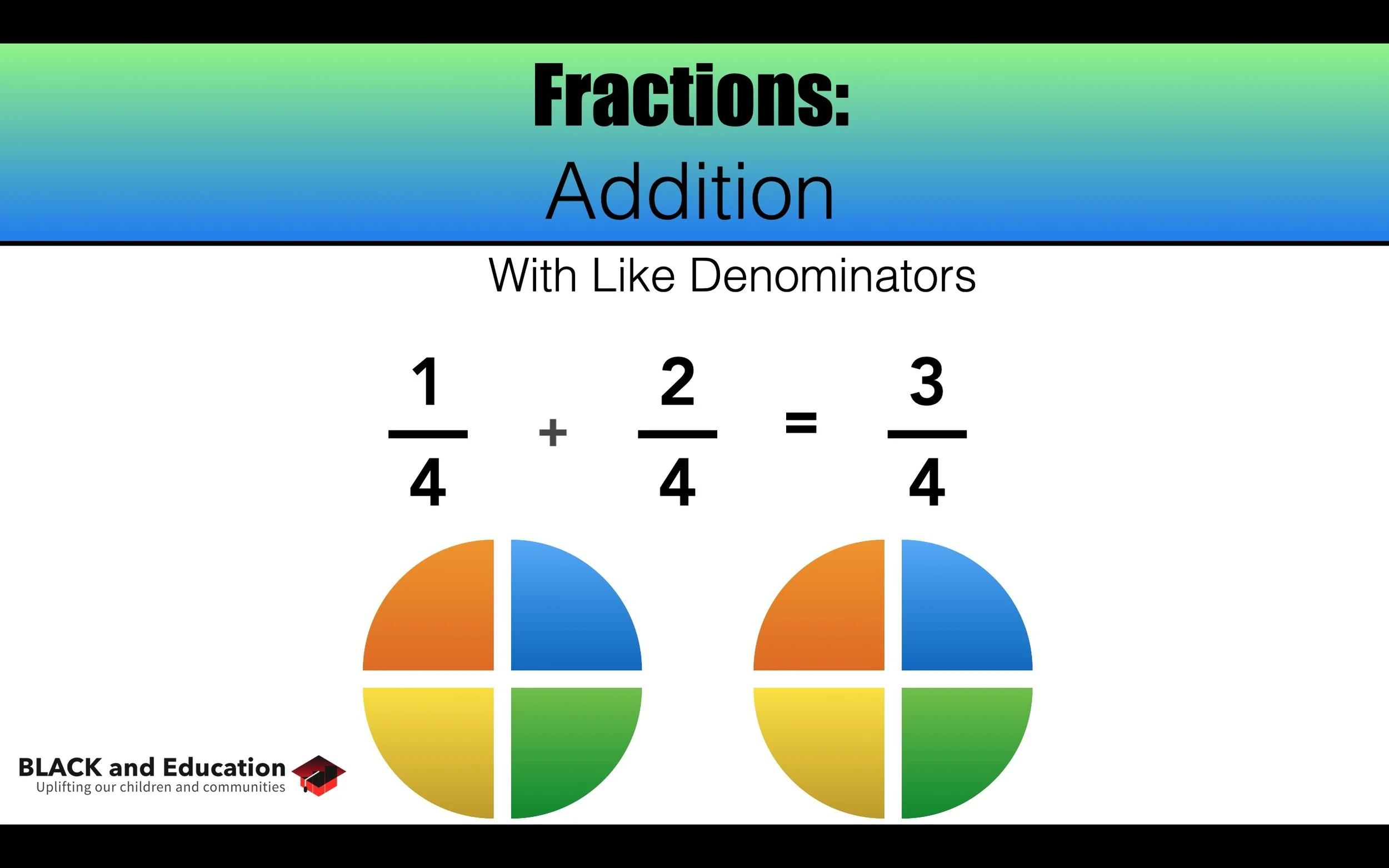
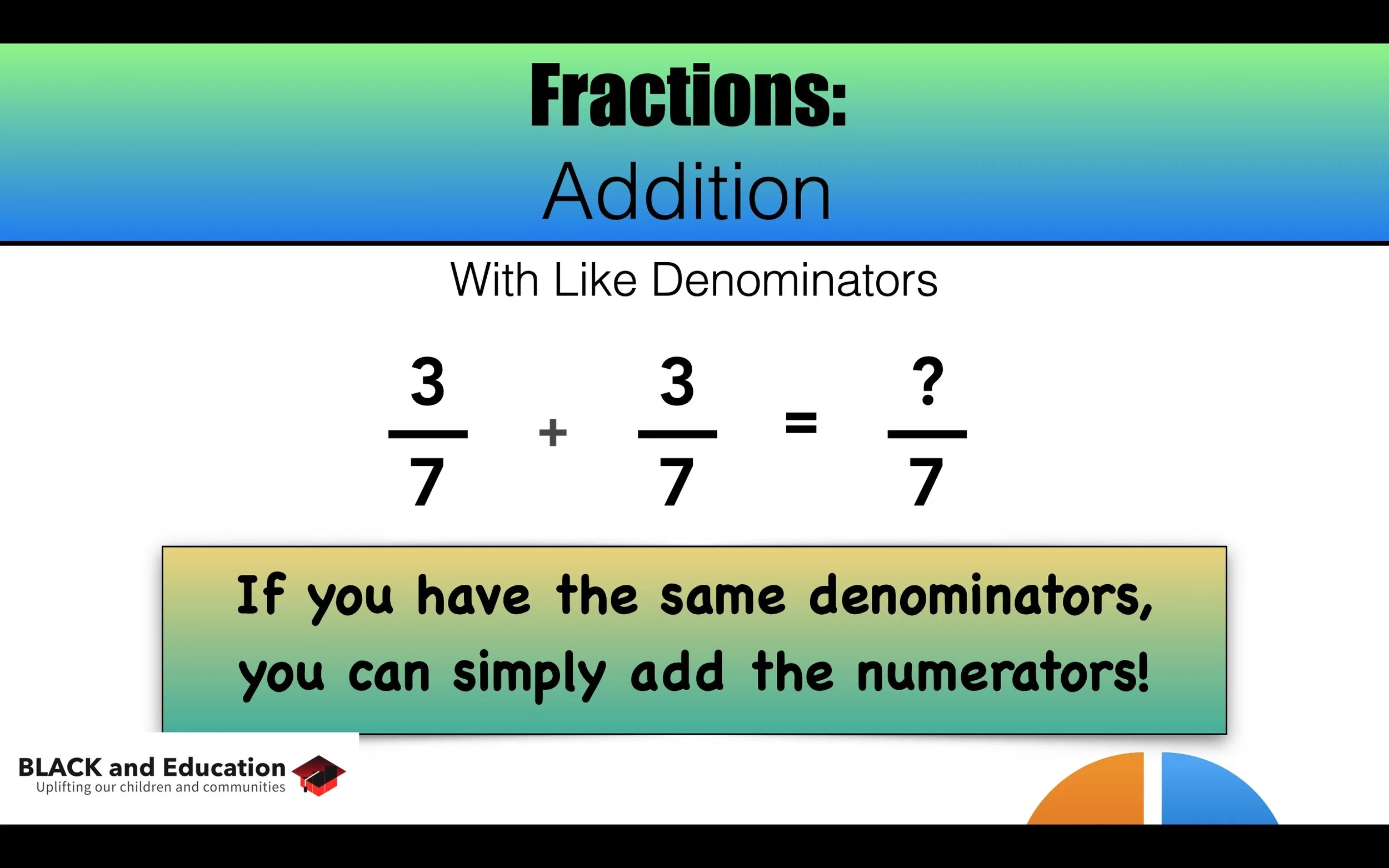
Adding Fractions with Like Denominators

If you gave 1/4 away to one friend today and then 2/4 away to two other friends tomorrow—you would have given away three-fourths (3/4) of your candy bar.

This brings us to a fundamental concept when it comes to fractions, if the denominators are the same, you can simply add the numerators in an addition problem.

This applies no matter what the denominator is:

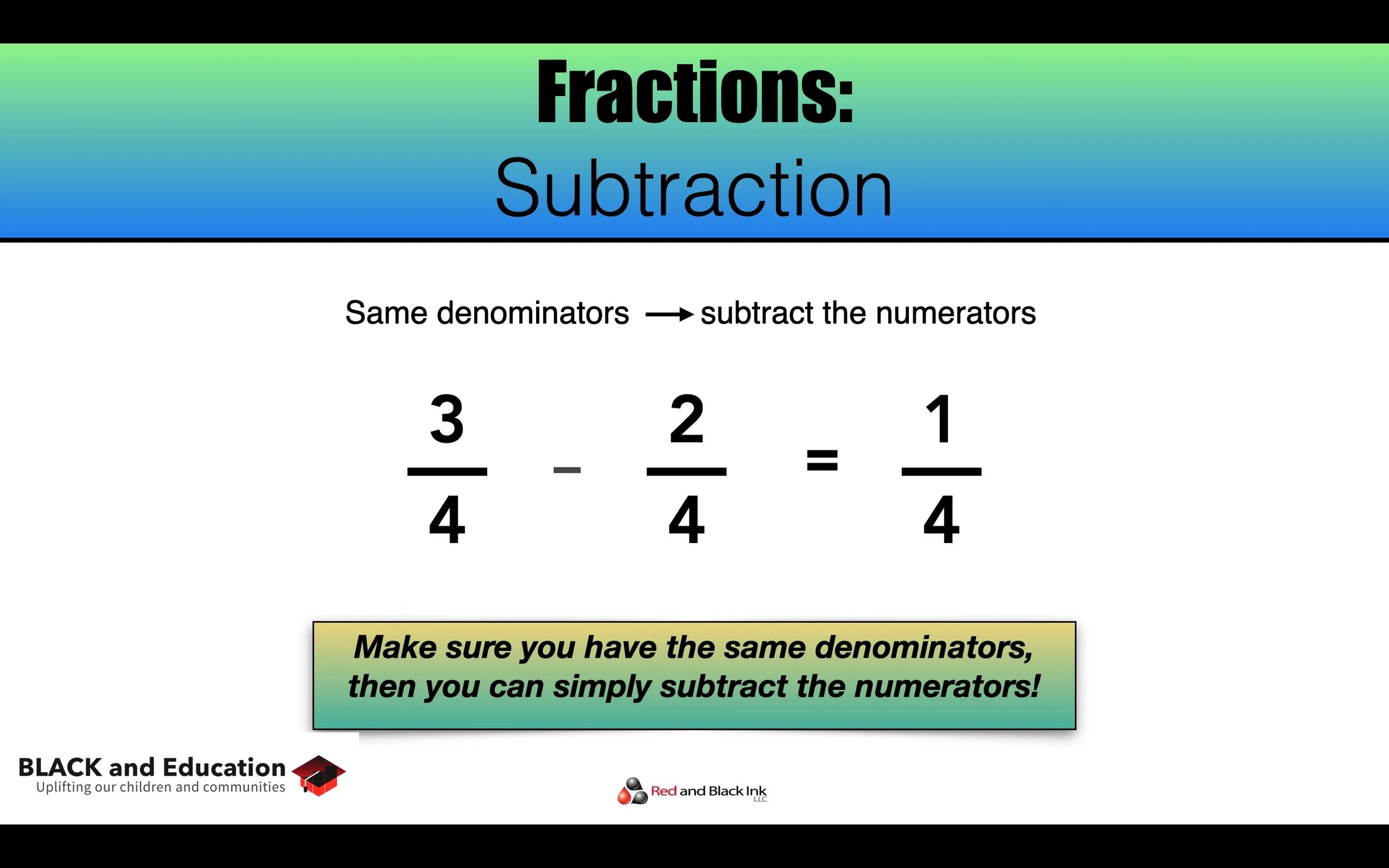
Subtraction with Like Denominators

The same thing is true with subtraction: when the denominators are the same simply subtract the numerators.

This again, applies to whatever the denominators are:

Adding and subtracting fractions with like denominators is straightforward.

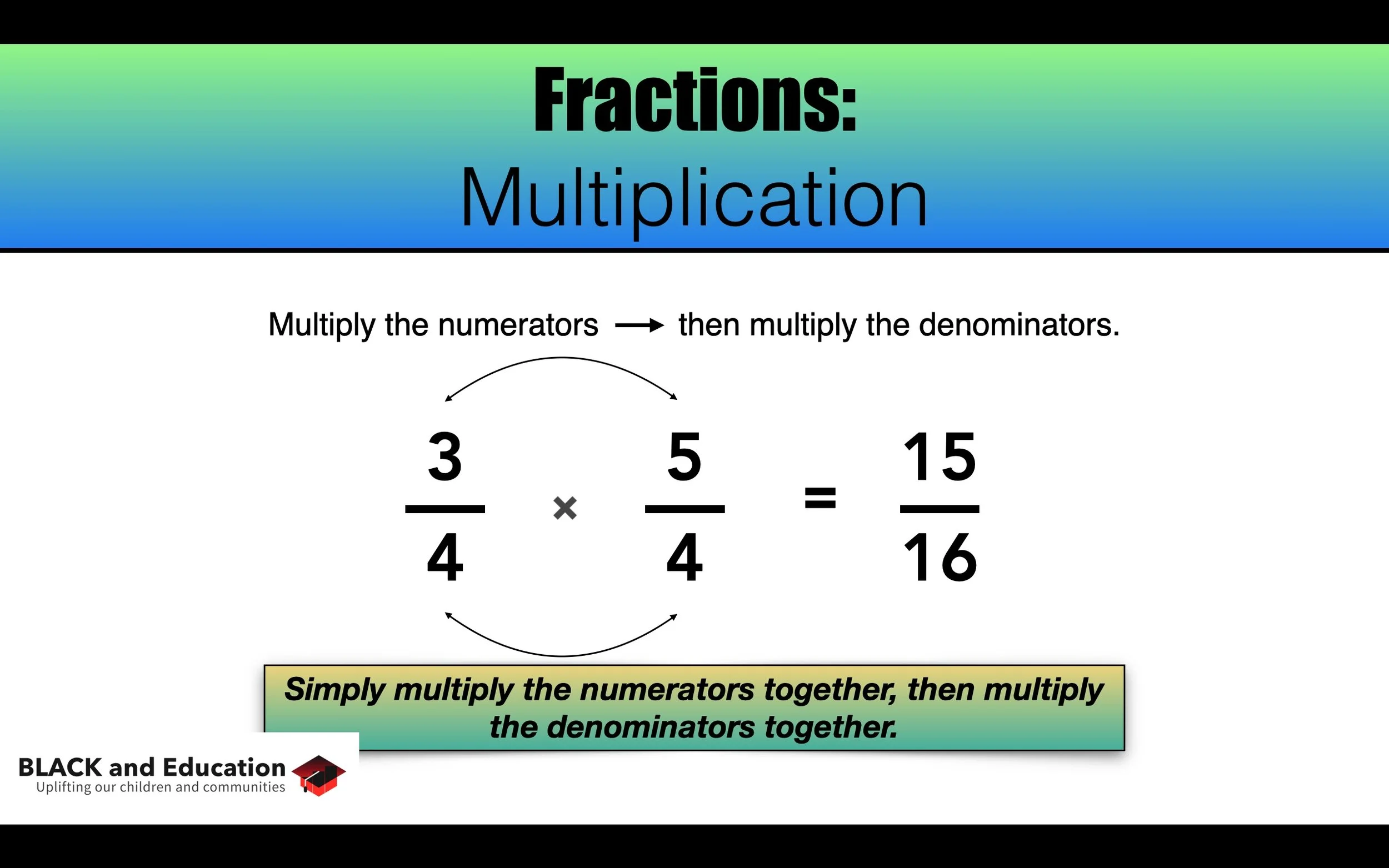
Multiplying Fractions

When multiplying fractions (whether they have the same denominators or not) is also straightforward—all you have to do is multiply the numerators, then multiply the denominators.

This is an important point to remember because many times you will have to take an expression, involving a fraction, and make it into a multiplication problem in order to solve it.

Sometimes, when you get the answer to a fraction problem, you will need to simplify it, but we will talk about that later.

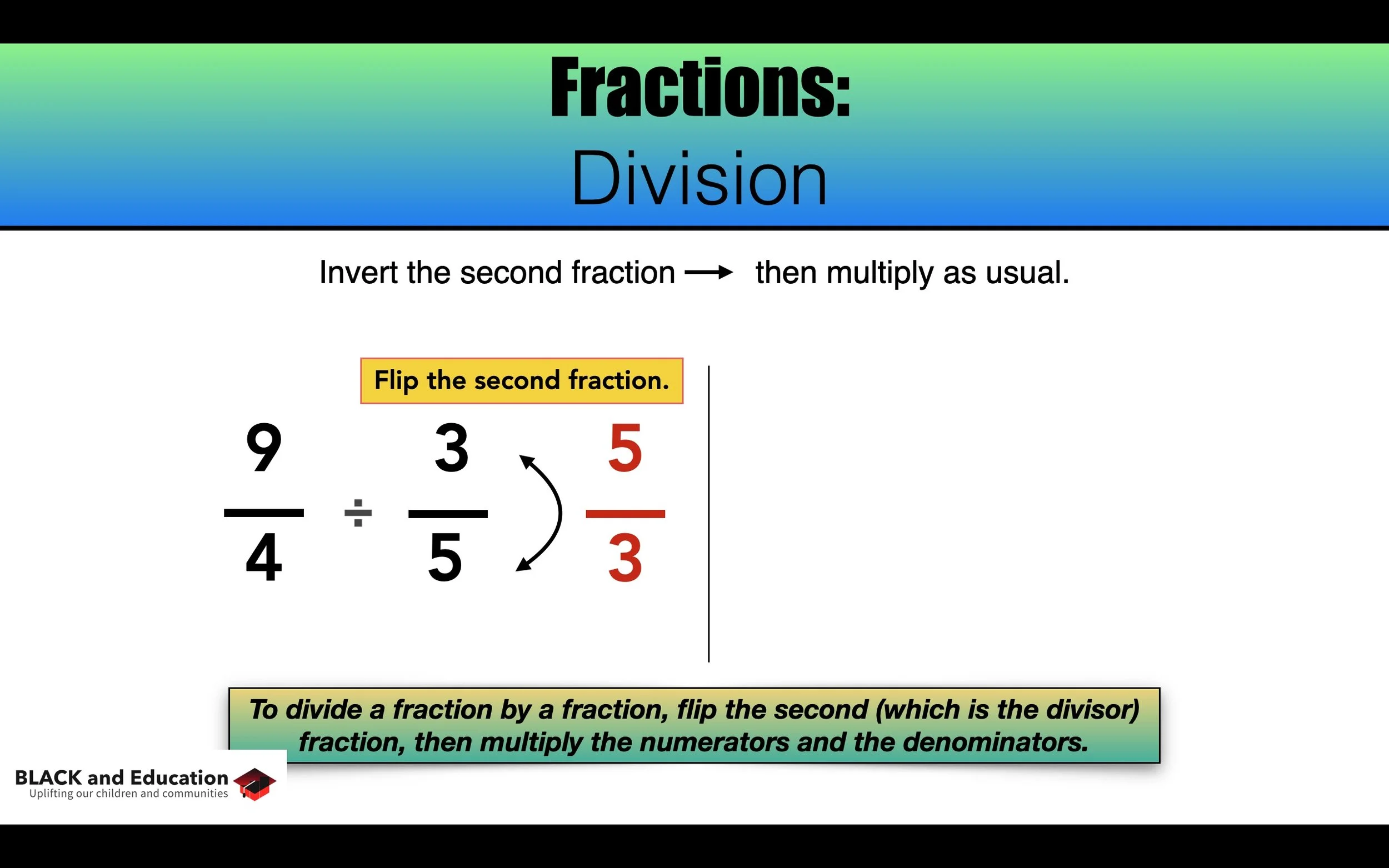
Dividing Fractions

When dividing a fraction by a fraction, you simply invert the second number (the divisor) to convert it to a multiplication problem, then solve. Take the problem below:

9/4 ÷ 3/5, because division is the opposite of multiplication—we can invert the second fraction and get the right answer by multiplying.

Multiply as usual-with fractions:

The above answer is in a slightly different form (the numerator is larger than the denominator), but we will address that shortly—let’s talk now about simplifying fractions.