Properties of Addition
Available on Amazon
This may also seem simple, but understanding the properties of addition and subtraction (as well as multiplication and division) will help you a great deal when you start to take algebra classes and beyond. So, let’s deal with a few important properties of addition, first.
Let’s make a quick note of vocabulary that may help you throughout math:
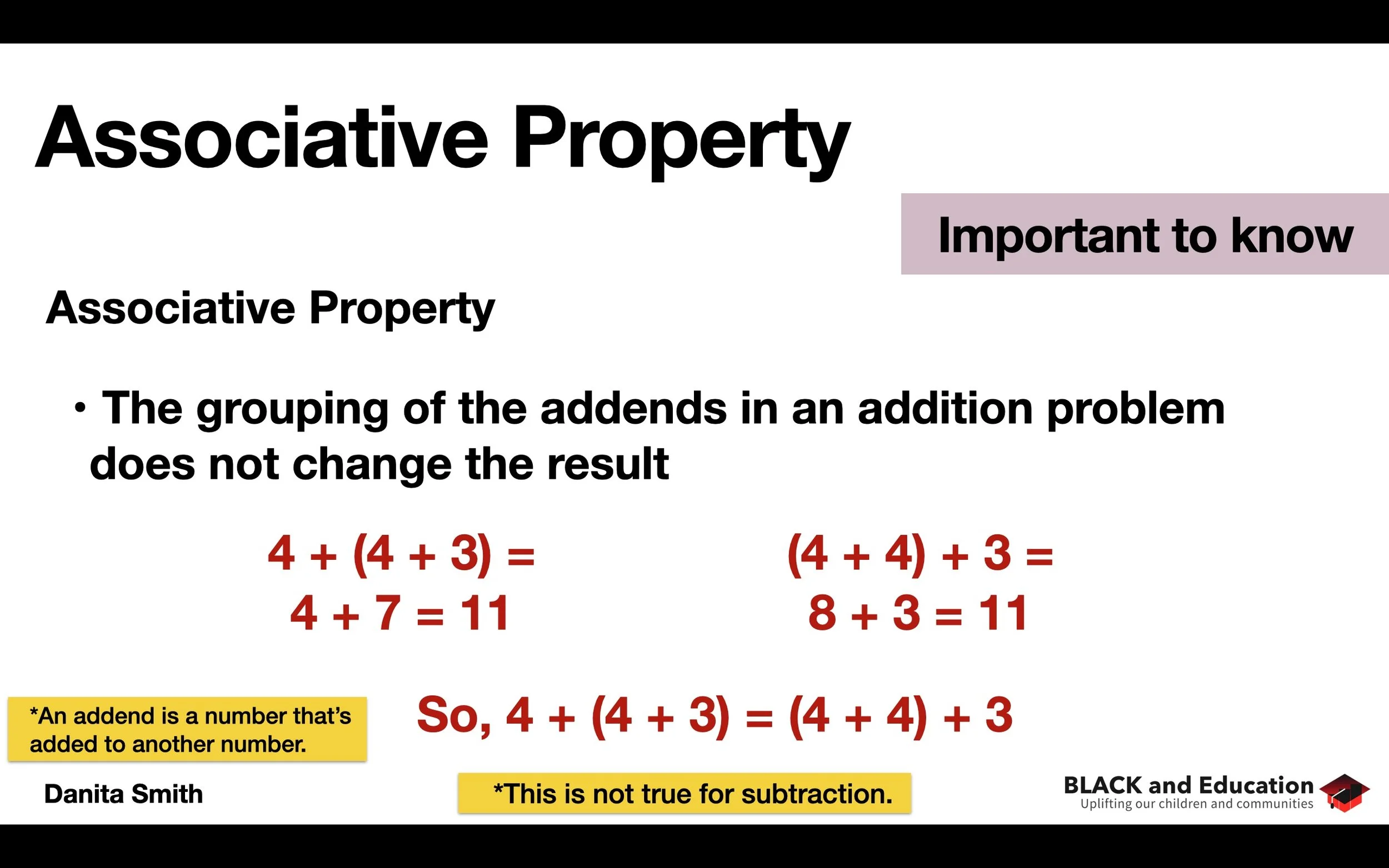
An addend is a number that is added to another number.
The sum is the result.
Addend + Addend = Sum
Grouping Doesn’t Matter
Associative Property of Addition
This property tells us that the way you group an addition problem with two or more addends, doesn’t matter—the result will be the same.
Ex. 1: (1 + 5) + 4 = 10 and (4 + 5) + 1 = 10
(1 + 5) + 4 = (4 + 5) + 1
Ex. 2: (9 + 7) + 6 = 22 and (7 + 6) + 9 = 22
(9 + 7) + 6 = (7 + 6) + 9
This is NOT true with subtraction.
Ex. 3: (22 - 9) - 8 = 5 and (8 - 9) - 22 = -23
5 is NOT equal to - 23
So, the way you group a subtraction problem DOES MATTER.
Order Doesn’t Matter
Commutative Property of Addition
This property says that the order of an addition problem does not change the result.
Ex. 1: 4 + 8 = 12 and 8 + 4 = 12
So, 4 + 8 = 8 + 4
This is NOT true with subtraction.
Ex. 2: 7 - 6 = 1 and 6 - 7 = -1
1 is NOT equal to -1
So, the order of a subtraction problem does matter.
Identity Property
Identity Property of Addition
This says that when 0 is added to any number, the sum (or result) is the number itself.
Ex. 1: 32,375 + 0 = 32,375
Summary
The order of the addends does not matter in an addition problem.
The way the addends are grouped together does not matter
Adding 0 to any number, equals the number itself