Multiplying and Dividing Fractions
Multiplying and dividing fractions really involve simple techniques.
When you multiply two fractions, you simply multiply the numerators together, then multiply the denominators.
When you divide fractions, you turn them into multiplication problems and solve them the same way.
To multiply the two fractions we see above 3/4 * 5/4, when multiply the numerators ( 3 * 5) and multiply the denominators (4 * 4). This will give us 15/16 (15 over 16 or fifteen sixteenths) as the answer.
Sometimes you will need to simplify your answer, but in this case, they share no common factors other than 1, so 15/16 is the final answer.
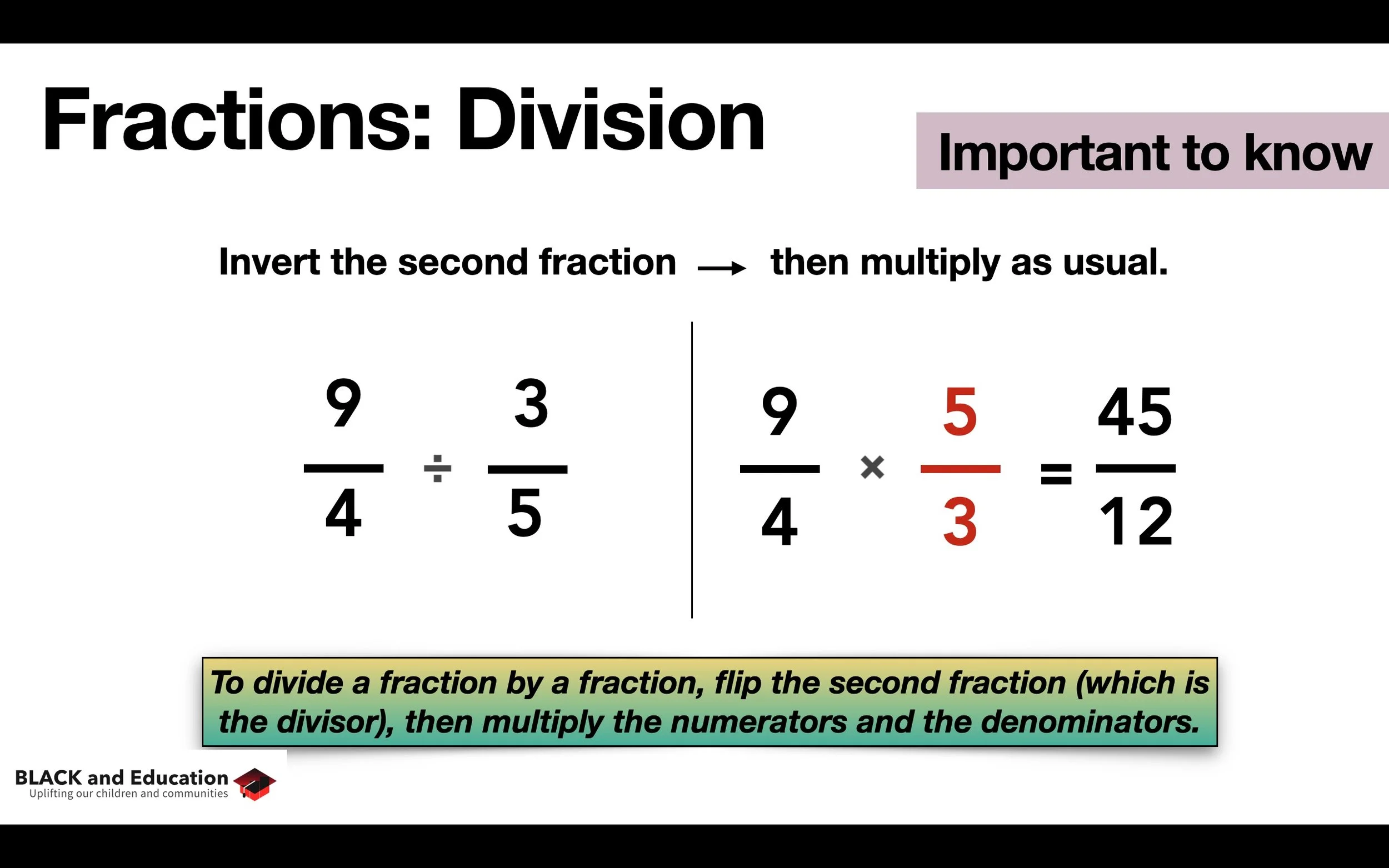
When it comes to division, you simply flip the divisor (the second fraction)—you invert it. Once you flip the divisor, you simply multiply the numerators (as you would do in a multiplication problem) multiply the denominators, as we explained for multiplication.
So, in this problem 9/4 ÷ 3/5…. you would flip the divisor so it would be 5/3 and the problem will now be 9/4 * 5/3.
Then you would multiply the numerators and finally multiply the denominators to get 45/12 as the final answer.
You will notice that 45/12 has a numerator that’s larger than its denominator—that means that it is an improper fraction and also a mixed number. We will talk about that in our next section.
Summary
When you multiply fractions, multiply the numerators, then the denominators to get the final answer.
When you divide fractions, flip the divisor (usually the section fraction) and follow the steps for multiplication.