Ten Things Your Child Should Know about Reading, Writing and Math

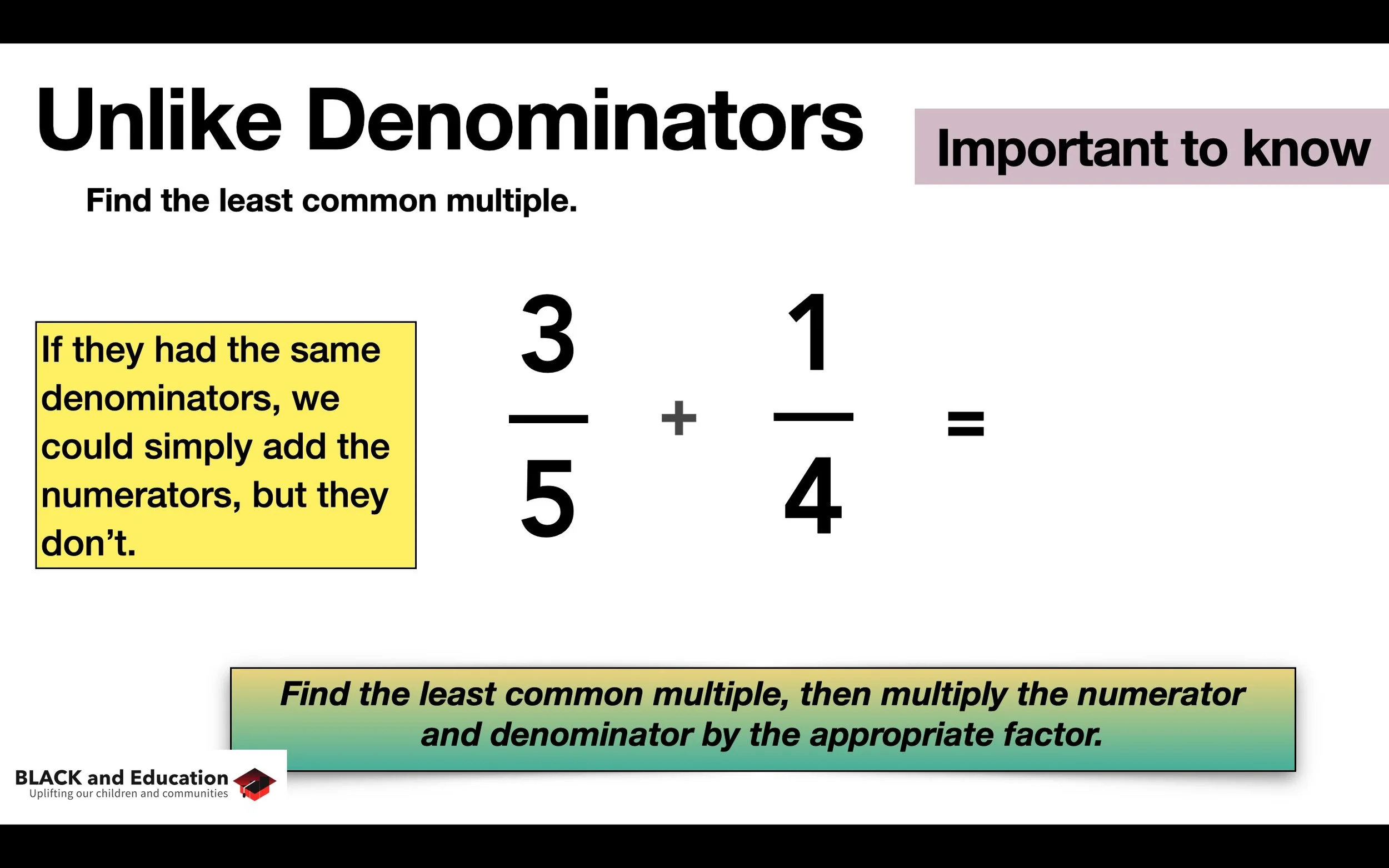
Here’s where finding the least common multiple comes in. You won’t always have fractions with the same denominator—that will come up in life and in math class. Well, what do you do when have to add or subtract two fractions with unlike denominators? If you had to multiply them, you just multiply the numerators together, then multiply the denominators. If you had to divide them, you just flip the divisor then multiply.

But, what about addition and subtraction?

Well, in order to add or subtract the fractions above we have to give them common denominators. To do this we find the least common multiple (LCM). In order to find the least common multiple we can multiply both numerators and both denominators by the factor that will give them their least common multiple. We are not changing the fraction because it’s just a ratio or percentage and since we are doing the same thing to the numerator and denominator in each fraction, the ratios (or fractions) remain the same.

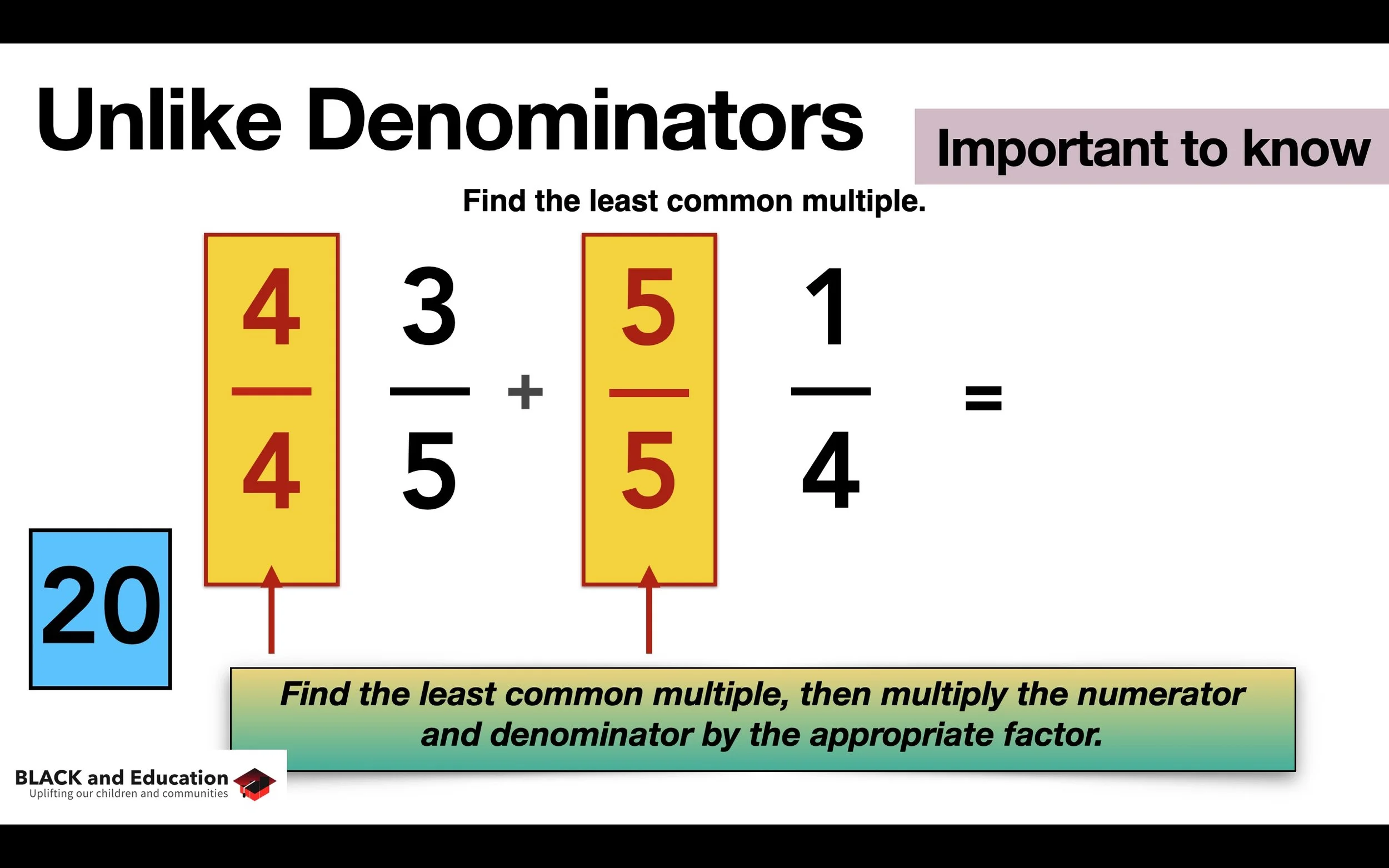
The least common multiple (the number that they both can divide into evenly) between the denominators, 4 and 5, is 20. I can easily see that in this situation because I have studied my multiplication facts well, so I can recognize that on sight (but if you had to you could write out their multiples until you came to the smallest one they have in common).

So, since it’s 20, I can multiply the numerator and denominator in the fraction 3/5, by 4 to get 12/20 as the new fraction on the left.

I can then multiply the numerator and denominator in the fraction 1/4, by 5 to get 5/20 as the new fraction on the right.

Now that I have 12/20 + 5/20, I can add these two fractions because they now have like denominators. The answer we be 17/20 and since there are no common factors other than 1 between 17 and 20, the fraction is in its simplest form and this is the final answer.

Summary

When you have tow fractions that have unlike denominators, you simply have to find the least common multiple of the denominators and multiply both the numerators and the denominators by the factors that will make them have the same denominator.
You don’t have to do this for multiplication because in multiplication you simply multiply the numerators together, then multiply the denominators together.

Ten Things Your Child Should Know about Reading, Writing and Math

Fractions: Unlike Denominators

Summary

When you have tow fractions that have unlike denominators, you simply have to find the least common multiple of the denominators and multiply both the numerators and the denominators by the factors that will make them have the same denominator.

You don’t have to do this for multiplication because in multiplication you simply multiply the numerators together, then multiply the denominators together.

Buy Our Books