Greatest Common Factor

Available on Amazon

Buy

Finding the Greatest Common Factor

Well, this is one of the handiest things you’ll do in math, in my opinion. Finding the greatest common factor (or common factors, in general) helps you simplify even the most complicated of problems, but let’s just talk about this concept first.

A factor, as you know, is a number that can divide evenly into its multiple without any remainders.

As you can imagine, different multiples can have many factors in common and many times you are working with more than just one number in your math problems, so finding the greatest common factor between two multiples can be useful.

As you can see above, 30 and 20 share common factors (such as: 5 and 10), but 10 is the greatest (or largest) factor they have in common, so it’s their greatest common factor.

Let’s look at 18 and 6. You may recognize that 6 is their greatest common factor because 6 is a factor of itself.

Sometimes it’s helpful to write out the factors that multiples have in common, in fact, it’s great to write out many things in math because how can you keep everything inside of your head?

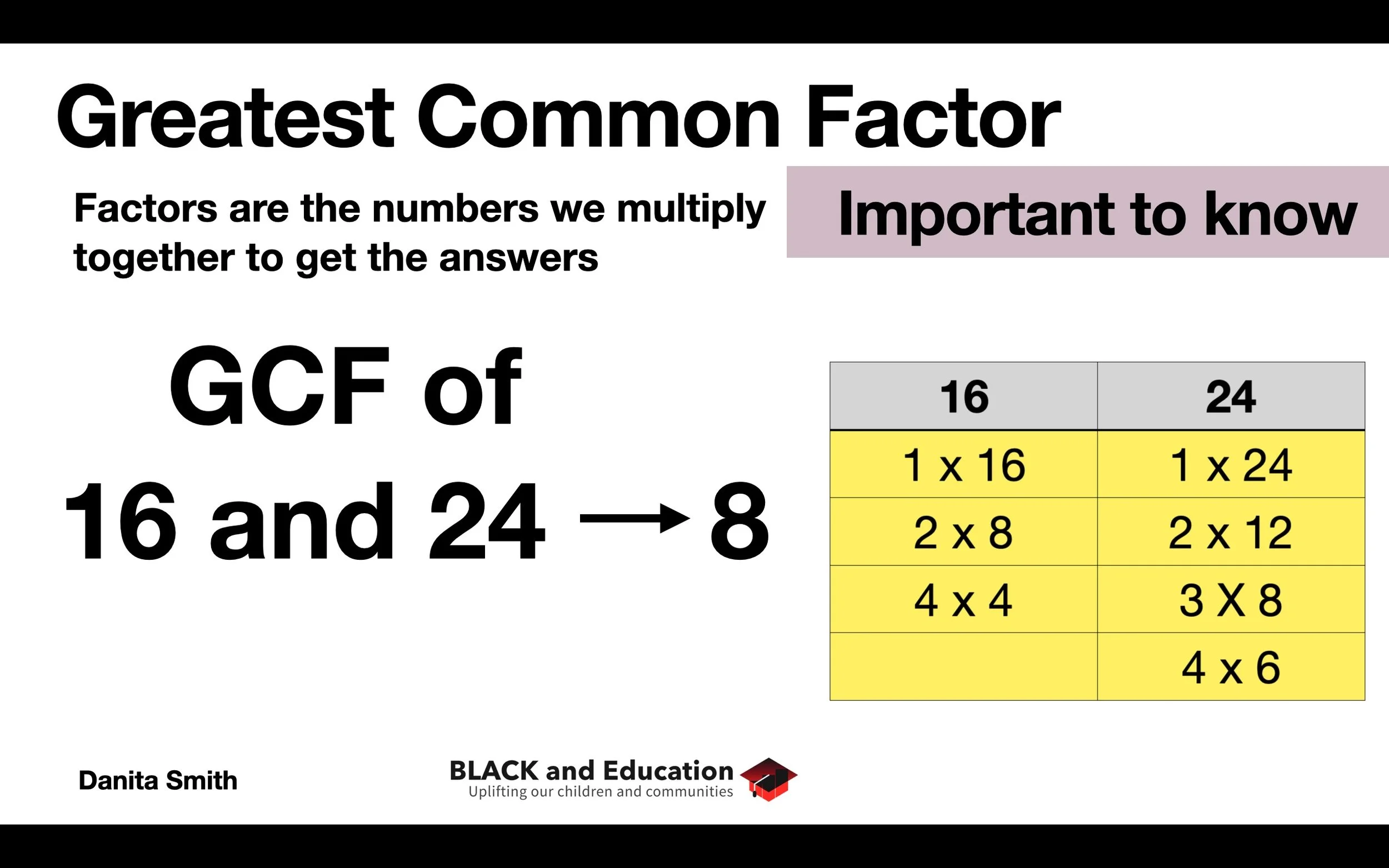
Here you can see that we’ve listed out the factors for 16 and 24 so that we can compare them.

When you do it this way, you will find that it’s easy to figure out the greatest common factor.

Summary

• The greatest common factor (GCF) is the largest factor that two numbers have in common.

• You will have to have a strong knowledge of your multiplication facts in order to find the greatest common factor of many problems.