Double-Digit Multiplication
Now it’s time to do slightly more advanced calculations with multiplication. You not only should be able to do long division by hand, but you should also be able to do longer multiplication problems by hand.
It will be easier for you if you can do multiplication problems that are longer when you need to, by hand, rather than doing everything with a calculator. You want to be able to understand what you are doing with a calculator before you start using it regularly.
As you know, each place in a number has an underlying value (or place value). When you start at the right you are in the ones place. When you move one place to the left, you are in the tens place. You have just moved up by a factor of 10.
So, when you multiply two numbers together, that have multiple digits, you have to make sure you line up your place values.
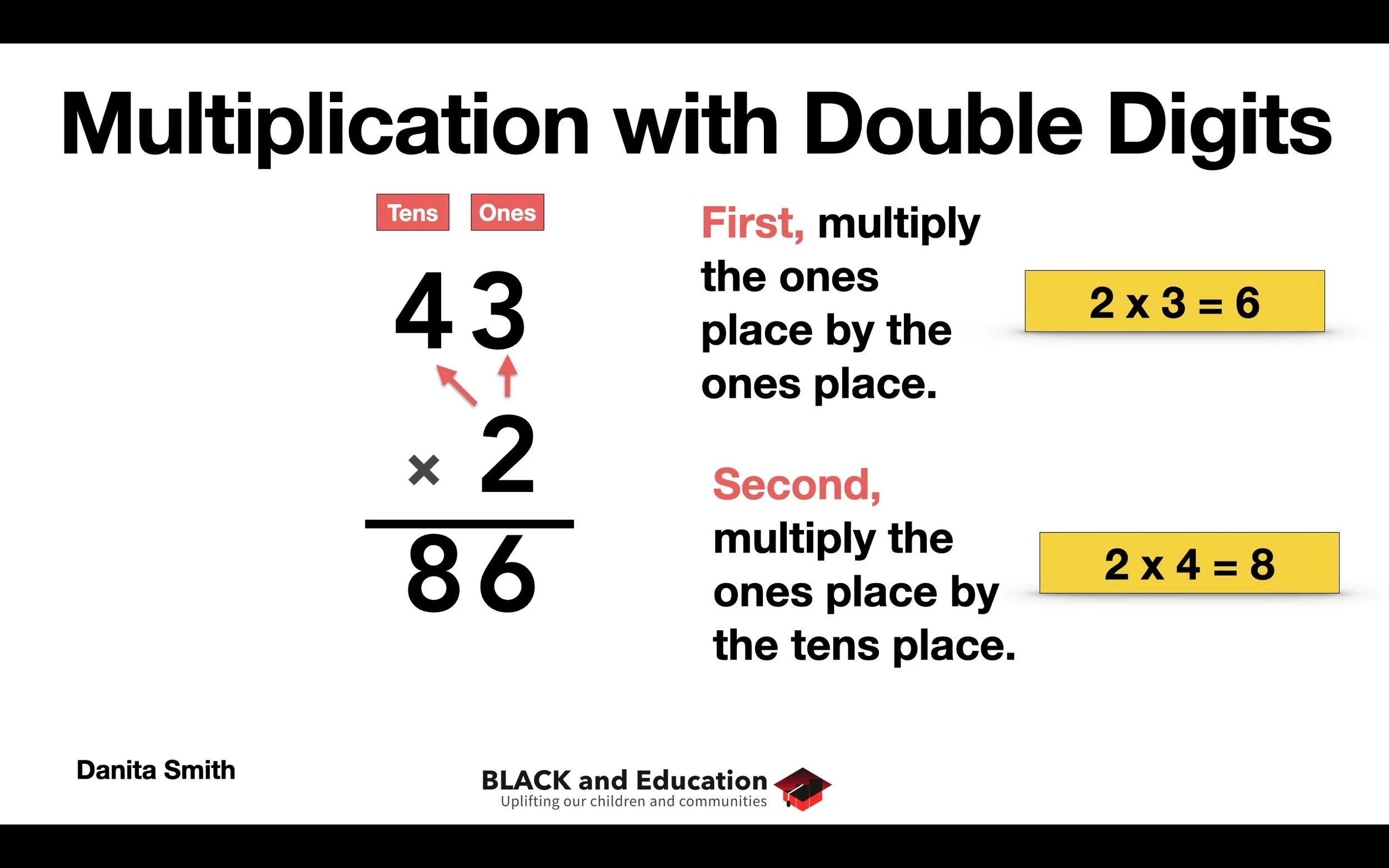
In the above example, 2 is in the ones place and 3 (in the number 43) is in the ones place. So, you line your problem up vertically and you make sure the ones place in the larger number is over the ones place in the smaller number.
43
x 2
86
You multiply the ones place on the bottom, by the ones place on top (2 x 3)…then the ones place on the bottom by the tens place on top (2 x 4). You will get (8 tens and 6 ones) 86 as an answer in this problem.
This is the simple process you follow to figure out these kinds of problems.
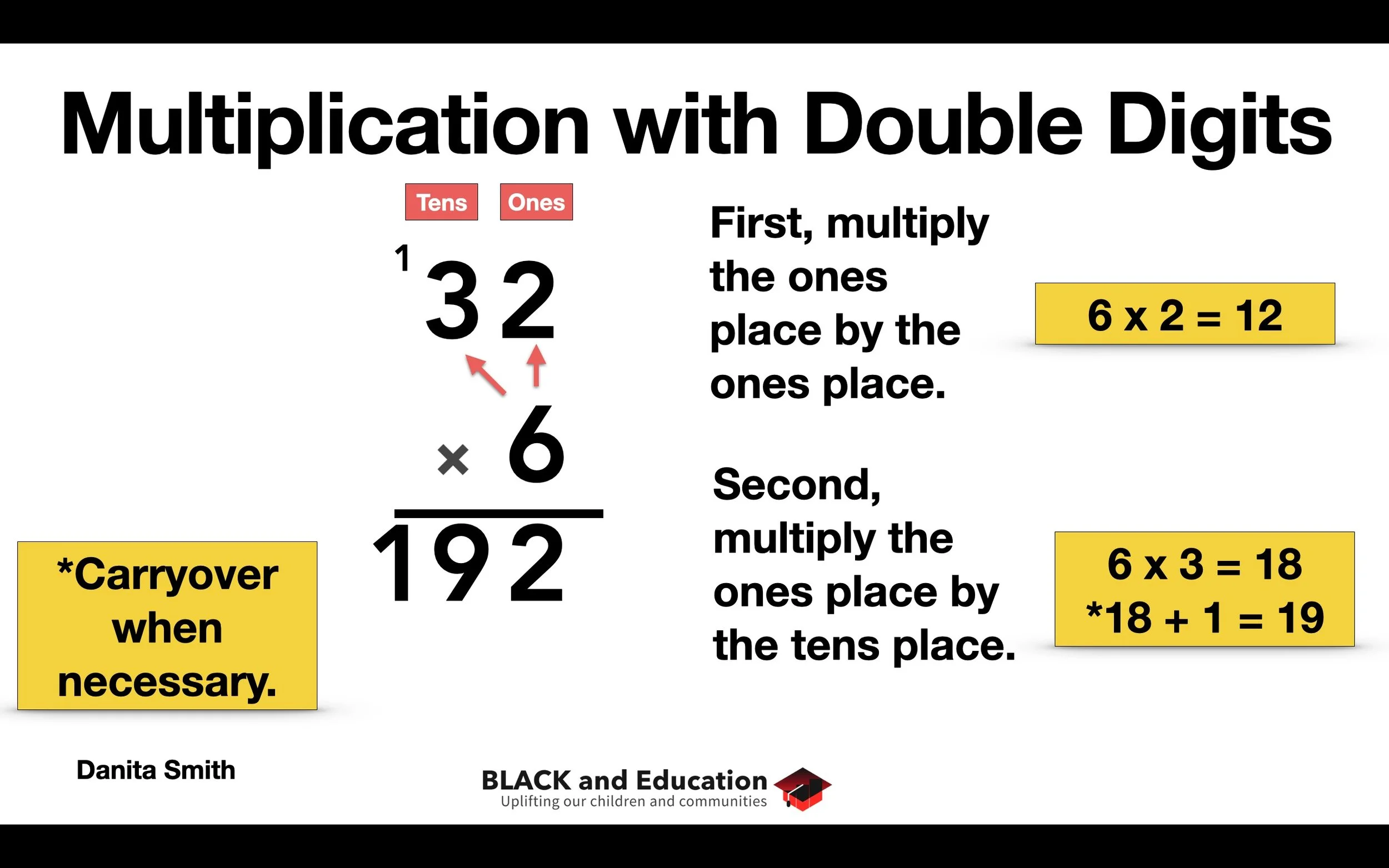
When you need to carryover a 10, let’s say, you carryover just as you would in an addition problem. You are carrying over one 10 in the problem above and adding it to the result of 6 x 3 (which will give you 19).
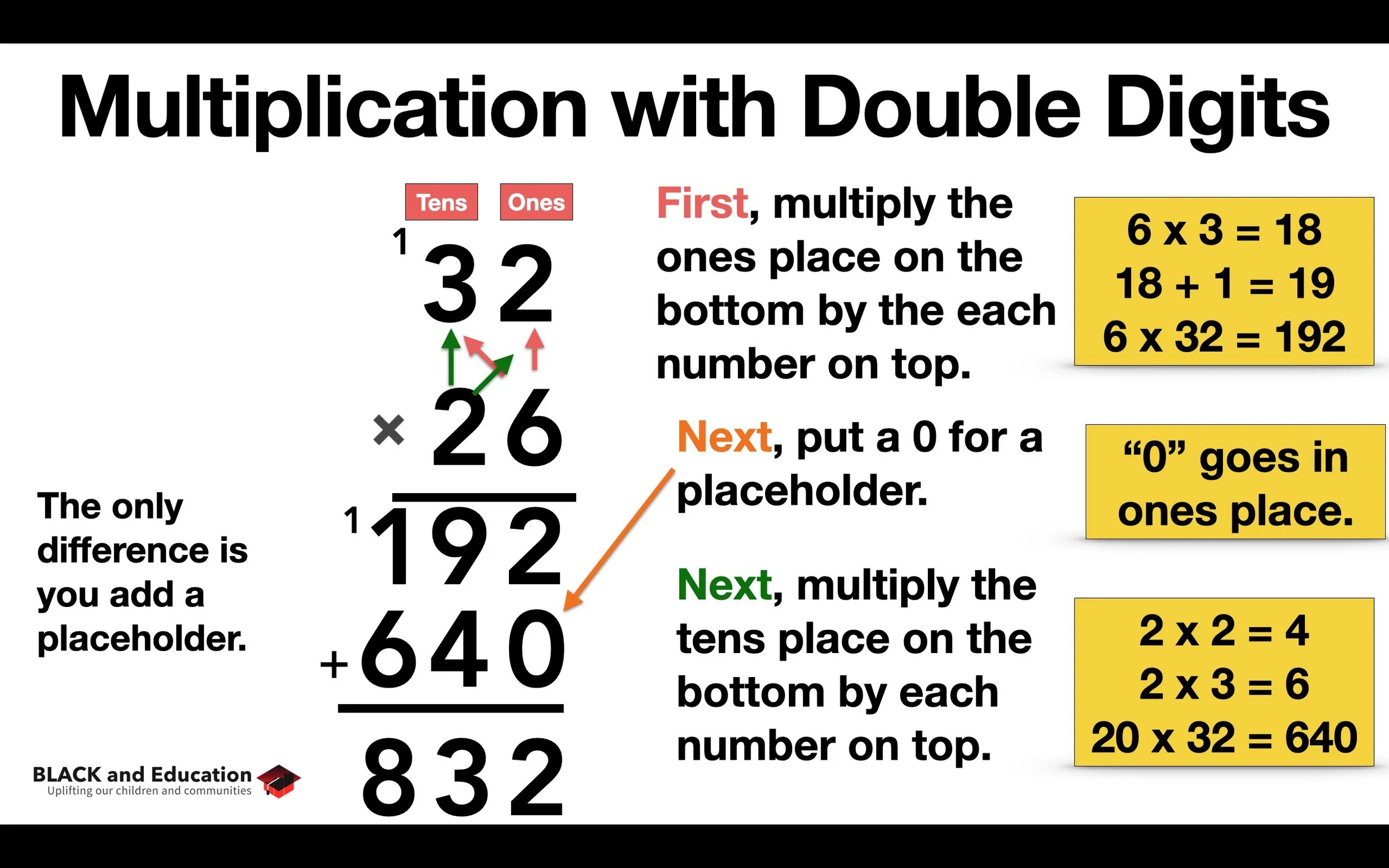
Now, when you have double digits in both factors, there is an additional step you must take.
When you have more than one digit in both factors you have to account for the extra place values, by adding a 0 in the bottom part of your problem where you add up the results.
For every additional place value you move to the left you add a 0 in the bottom part where you add up the results.
So, once you finish multiplying out 6 x 32 and figure out that it’s 192, you add a 0 under the 2 at the bottom and begin multiplying two times 32 at the top—to account for the 20 in 26. This will give you 640, and when you add 192 + 640 you will get 832 as the answer. This way you can add any number that has multiple digits in each factor, simply by lining up the place values and adding an additional 0 at the bottom when you move to the left in the problem.
Summary
When multiplying factor with double digits, make sure you line up the place values.
Also, account for the moves to the left (to higher place values) by adding a 0 as appropriate at the bottom where you add up your results.